Embark on an intellectual journey into the realm of worksheet arithmetic and geometric sequences, where we unravel the intricacies of these mathematical progressions. Delving into their unique characteristics, formulas, and applications, we uncover the power and versatility of these sequences in shaping our understanding of the world around us.

In this comprehensive exploration, we will meticulously examine the defining traits of arithmetic and geometric sequences, unraveling their patterns and properties. Through practical examples and insightful explanations, we will master the art of calculating their terms and uncover their significance in various fields.

Arithmetic and Geometric Sequences: Worksheet Arithmetic And Geometric Sequences

Sequences are ordered lists of numbers that follow a specific pattern. Arithmetic and geometric sequences are two types of sequences that are commonly used in mathematics and have various applications in real-world scenarios.

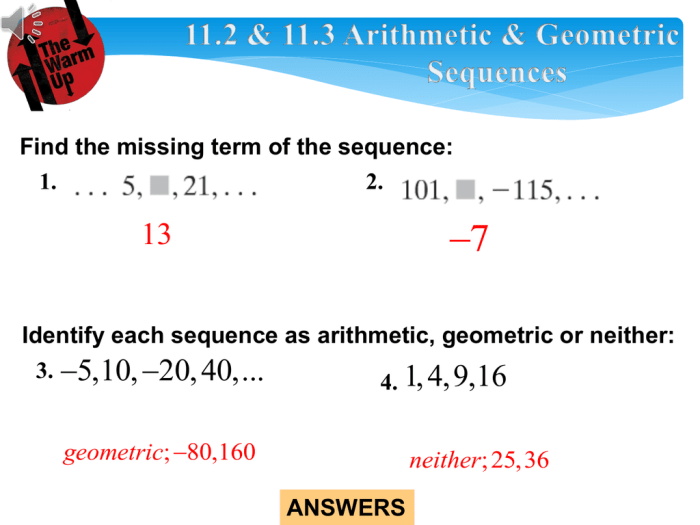
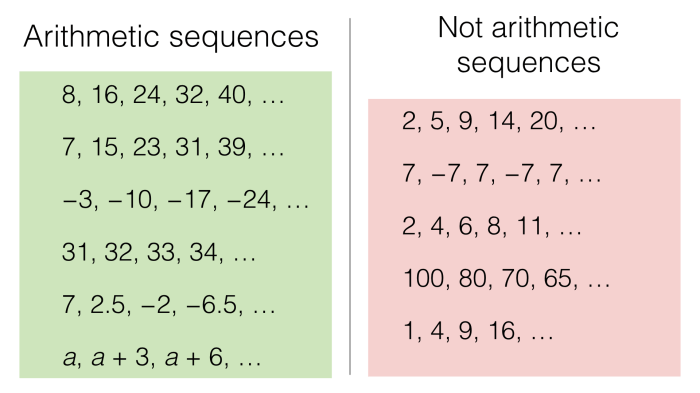
Arithmetic sequences are characterized by a constant difference between consecutive terms, while geometric sequences have a constant ratio between consecutive terms.

Arithmetic Sequences, Worksheet arithmetic and geometric sequences

The formula for finding the nth term of an arithmetic sequence is:

a _n= a ₁+ (n – 1)d

where a _nis the nth term, a ₁is the first term, n is the term number, and d is the common difference.

For example, the sequence 2, 5, 8, 11, … is an arithmetic sequence with a common difference of 3.

Arithmetic sequences have several properties, including:

• The sum of any two consecutive terms is equal to the sum of the first and last terms.
• The average of any two consecutive terms is equal to the middle term.
• The sum of the first n terms is equal to n/2(a ₁+ a _n).

Geometric Sequences

The formula for finding the nth term of a geometric sequence is:

a _n= a ₁– r ^{(n- 1)}

where a _nis the nth term, a ₁is the first term, n is the term number, and r is the common ratio.

For example, the sequence 2, 4, 8, 16, … is a geometric sequence with a common ratio of 2.

Geometric sequences have several properties, including:

• The ratio of any two consecutive terms is equal to the common ratio.
• The product of any two consecutive terms is equal to the square of the middle term.
• The sum of the first n terms is equal to a ₁(1 – r ⁿ)/(1 – r).

Applications of Arithmetic and Geometric Sequences

Arithmetic and geometric sequences have numerous applications in real-world situations, such as:

• Finance: Calculating interest rates, loan payments, and compound interest.
• Physics: Modeling projectile motion, radioactive decay, and population growth.
• Computer science: Generating Fibonacci numbers, analyzing algorithms, and compressing data.

The choice of using an arithmetic or geometric sequence depends on the specific application and the pattern of the numbers involved.

User Queries

What is the key difference between arithmetic and geometric sequences?

In arithmetic sequences, the difference between consecutive terms is constant, while in geometric sequences, the ratio between consecutive terms is constant.

How do I find the nth term of an arithmetic sequence?

Use the formula: nth term = first term + (n-1) – common difference.

What are some real-world applications of geometric sequences?

Geometric sequences are used in population growth models, radioactive decay, and compound interest calculations.